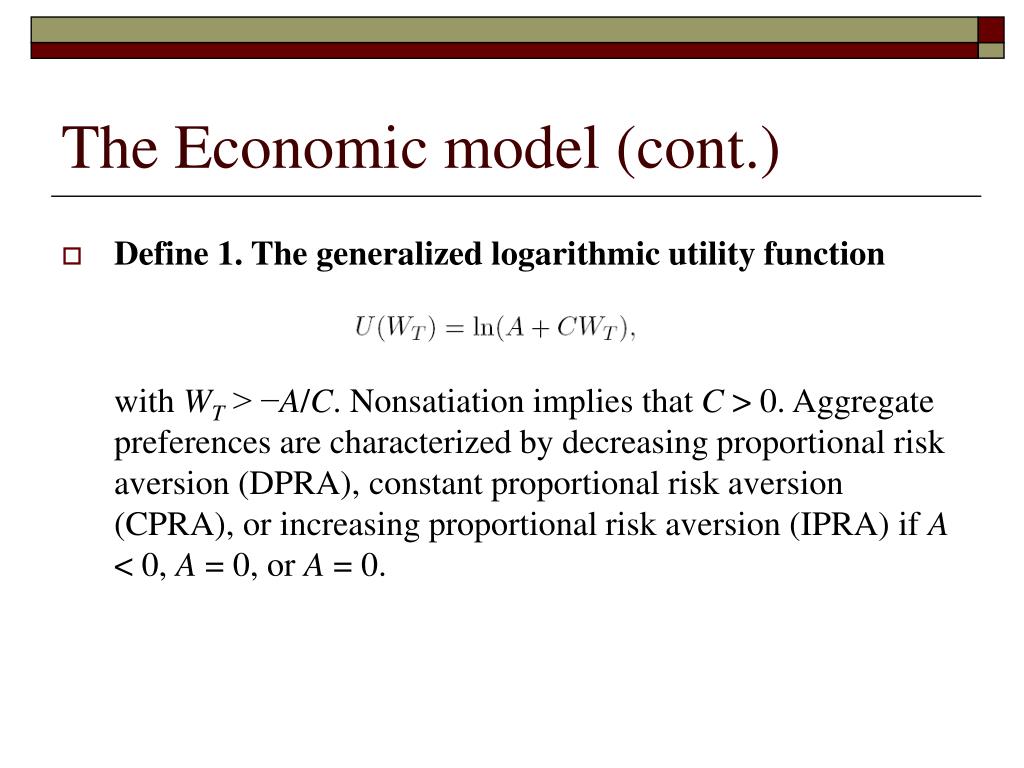
Lognormal refers to the facts that changes in the natural, or base e, logarithm of the stock price are assumed to be normally distributed, and that the resulting distribution of possible stock prices at a particular time is a lognormal distribution.
What is the use of lognormal distribution in statistics?
Lognormal is extremely useful when analyzing stock prices. As long as the growth factor used is assumed to be normally distributed (as we assume with the rate of return), then the lognormal distribution makes sense. Normal distribution cannot be used to model stock prices because it has a negative side, and stock prices cannot fall below zero.
Why do stock prices follow a lognormal distribution?
An important point to note is that when the continuously compounded returns of a stock follow normal distribution, then the stock prices follow a lognormal distribution. Even in cases where returns do not follow a normal distribution, stock prices are better described by a lognormal distribution.
Can normal distribution be used to model stock prices?
Normal distribution cannot be used to model stock prices because it has a negative side, and stock prices cannot fall below zero. Another similar use of the lognormal distribution is with the pricing of options . The Black-Scholes model—used to price options—uses the lognormal distribution as its basis to determine option prices .
How do you find the lognormally distributed rate of return?
If the rate of return r is continuously compounded then the future stock price can be expressed as: S t = S 0*EXP(r) S 0 is a known quantity and is a constant. This expression is the same as Y = exp(X). Therefore, if r is normally distributed, the stock price will be lognormally distributed.
Do stock prices follow a lognormal distribution?
When the returns on a stock (continuously compounded) follow a normal distribution, the stock prices follow a lognormal distribution. Note that even if returns do not follow a normal distribution, the lognormal distribution is still the most appropriate model for stock prices.
Why is the lognormal distribution a good fit to describe the behavior of a stock price?
A lognormal distribution is commonly used to describe distributions of financial assets such as share prices. A lognormal distribution is more suitable for this purpose because asset prices cannot be negative.
Under what condition log normal distribution is used?
The lognormal distribution is used under these conditions: Upper and lower limits are unlimited, but the uncertain variable cannot fall below the value of the location parameter. Distribution is positively skewed, with most values near lower limit. Natural logarithm of the distribution is a normal distribution.
What is true regarding normal and log normal distributions?
The lognormal distribution differs from the normal distribution in several ways. A major difference is in its shape: the normal distribution is symmetrical, whereas the lognormal distribution is not. Because the values in a lognormal distribution are positive, they create a right-skewed curve.
Why do we use log normal distribution?
The log-normal distribution curve can therefore be used to help better identify the compound return that the stock can expect to achieve over a period of time. Note that log-normal distributions are positively skewed with long right tails due to low mean values and high variances in the random variables.
Why is the lognormal distribution important?
Lognormal distribution plays an important role in probabilistic design because negative values of engineering phenomena are sometimes physically impossible. Typical uses of lognormal distribution are found in descriptions of fatigue failure, failure rates, and other phenomena involving a large range of data.
What are the two parameters of a lognormal distribution?
The lognormal distribution has two parameters, μ, and σ. These are not the same as mean and standard deviation, which is the subject of another post, yet they do describe the distribution, including the reliability function.
Why are stock prices Lognormally distributed?
While the returns for stocks usually have a normal distribution, the stock price itself is often log-normally distributed. This is because extreme moves become less likely as the stock's price approaches zero. Cheap stocks, also known as penny stocks, exhibit few large moves and become stagnant.
What is the range of lognormal distribution?
The case where θ = 0 and m = 1 is called the standard lognormal distribution....1.3. 6.6. 9. Lognormal Distribution.Meane^{0.5\sigma^{2}}Range0 to \inftyStandard Deviation\sqrt{e^{\sigma^{2}} (e^{\sigma^{2}} - 1)}Skewness(e^{\sigma^{2}}+2) \sqrt{e^{\sigma^{2}} - 1}Kurtosis(e^{\sigma^{2}})^{4} + 2(e^{\sigma^{2}})^{3} + 3(e^{\sigma^{2}})^{2} - 33 more rows
Why the mean of the lognormal distribution is larger than median?
The mean being greater than the median is another sign that the lognormal distribution is skewed right.
How do you find the expected value of lognormal?
0:0014:33Expectation of Log-Normal Variable - YouTubeYouTubeStart of suggested clipEnd of suggested clipAnd we know that the expectation of X is equal to its mean mu. But Y here is log normallyMoreAnd we know that the expectation of X is equal to its mean mu. But Y here is log normally distributed. So that is because y equals e to the power of X.
Can the mean of a lognormal distribution be negative?
All values in a lognormal distribution are positive. Negative values and zeroes are not possible in a lognormal distribution.
How is normal distribution used in stock market?
A normal distribution is a sample with an equal distribution above and below average. New Delhi (OpeningBell.in): When you invest in a stock, you expect to get more returns than the money you put in. Analysts and investors often use a normal probability distribution when analyzing the returns of a stock.
Why do we use log returns in finance?
log values effectively captures the compounding effect. the compounding of returns of stocks can be easily understand by assuming the normal distribution. Natural log value is the more appropriate measure for computing the change in variable which follows normal distribution.
Why does Black Scholes use lognormal distribution?
Black-Scholes assumes stock prices follow a lognormal distribution because asset prices cannot be negative (they are bounded by zero).
What is the distribution of stock prices?
Distribution stock refers to a large blocks of a security that are carefully sold into the market gradually in smaller blocks so as to inundate the market with sell orders for the security and driving down its price. Traders also refer to the dynamic of securities being sold this way as simply "distribution."
Why is there a systematic component to the random walk process?
There is a systematic component due to the fact that stock prices tend to increase over time. The random walk part of the process represents deviations up and down from that trend.
What is the volatility of a stock?
For example, consider a stock for which the expected increase in value per year is 10% and the volatility of the stock price is 30%. The volatility of a stock price is a measure of its variability; for a detailed discussion of volatility please review the white paper on this topic. The following graph shows the probability distribution of possible prices 1, 2, and 3 years from now if the price of the stock follows a lognormal random walk with drift:
What is random walk?
A random walk process is one in which the change in value over any time interval is independent of any changes that have occurred in preceding time intervals, and the size and direction of the changes in value are in some sense random. For stock prices, the applicability of a random walk is based on the assumption that the stock market is ...
How to salvage a normal model?
One can try to salvage a normal model by introducing relative changes that are expressed as percentages of the current stock price. There are problems with this as well, however. One is that prices can still go negative. If we ignore drift, a price change of -125% will be just as likely as a price change of +125% if we assume that the changes are normally distributed. Yet a deviation of more than 100% in the negative direction is impossible, since it will lead to a negative price, while a deviation of more than 100% in the positive direction is quite plausible; there is no reason that the stock price cannot more than double.
What does negative Ln mean?
All ln’s greater than negative infinity correspond to positive numbers; a negative ln just means the number is less than 1. Thus, by modeling the ln’s of prices, we guarantee that prices remain positive, though they can become quite small.
What is the difference between % change and ln?
The Change in ln and Change in ln Expressed as % columns consist of the same two columns of numbers, just expressed differently. For example, 0.010 expressed as a percentage is 1.0%. The Corresponding % Change columns show what the resulting percentage change is for a given change in ln. For example, if the ln of a number increases by 0.100, the number increases by 10.5%.
Is a lognormal distribution normal?
Readers who have taken a statistics course will recognize that the above probability distributions resemble normal distributions, and in fact they are indeed normal distributions. Thus, the probability distributions in the preceding graph are lognormal distributions.
Why is lognormal distribution used?
A lognormal distribution is commonly used to describe distributions of financial assets such as share prices. A lognormal distribution is more suitable for this purpose because asset prices cannot be negative.
What happens to stock price if R is normally distributed?
Therefore, if r is normally distributed, the stock price will be lognormally distributed.
What is the opposite of logs?
Exp (X) or e x is the opposite of taking logs. If we take log on both side, we will have ln y = X
Does the skewed distribution have a long tail?
The distribution is skewed to the right, i.e., it has a long right tail.
Is lognormal distribution related to normal distribution?
The concept of lognormal distribution is very closely related to the concept of normal distribution.
What is lognormal distribution?
From the definition of a Lognormal distribution, if log (S t) is normally distributed, then S t must be lognormally distributed. Thus, under this model, the price of a stock will be lognormal.
What is the most common model for the price of a non-dividend paying stock?
The most common model for the price of a non-dividend paying stock comes from a model of the return of a stock , which is assumed to follow an Ito process, as follows (see, for example, Hull (1993), Chapter 9 and Wilmott (2001) ):
What is mean reversion in stock?
An extension to this model is to consider mean reversion, which means that the stock price tends to move back towards the expected return as a function of how far away it is at any moment from that expected return. The section Stock price with mean reversion extends the above model to include mean reversion.
Why are prices always positive?
Historically, normally distributed prices where first considered (models such as an arithmetic Brownian motion), but Samuelson introduced the geometric Brownian motion to avoid negativity. Black and Scholes built upon this insight. Hence, prices are always positive but returns may be negative.
Is S t log normal?
Note that both, the stock price and its returns are stochastic processes and hence " S t is log-normally distributed" really means that the random variable S t ( ω) follows a log-normal distribution for each fixed time point t > 0.
Is stock price log normal?
In reality, neither are stock prices log-normally distributed nor are returns normally distributed. More sophisticated models drop this assumption. For instance, returns are more peaked and have fatter tails than a normal distribution would suggest.
Can stock prices be negative?
Stock prices cannot be negative which means that they are not normally distributed due to the fact they cannot be negative as result of this stock prices behave similarly to exponential functions. To transform this exponential values back to a normally distributed variable, you need to take the natural logarithm, and therefore can take a lognormal value and distribution.